Places To Go, and Ways to Get There
Here are two versions of the Königsberg map. On the left is a smaller version of the map you've already seen, and on the right is the version Euler used in his 1735 proof.
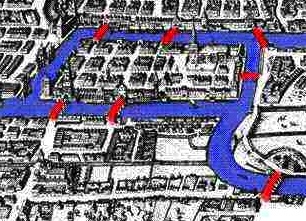
Traditional map
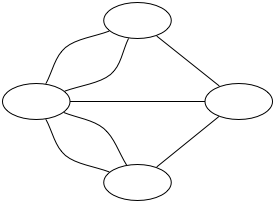
Euler's version
Here's what I think is so mind-bendingly cool about this: Euler threw away all the information that didn't matter to the problem.

If all you're concerned with is which bridges you cross, then for all intents and purposes, each land mass can be represented by a point, and each bridge is just a line between two points.
Which is why I say you can think of graph theory as the study of places to go, and ways to get there.
- About This Site
- Git Makes More Sense When You Understand X
- Example 1: Kent Beck
- Example 2: Git for Ages 4 and Up
- Example 3: Homeomorphic Endofunctors
- Example 4: LSD and Chainsaws
- The Internet Talks Back!
- Graph Theory
- Seven Bridges of Königsberg
- Places To Go, and Ways to Get There ←HEAD
- Nodes and Edges
- Attaching Labels to Nodes
- Attaching Labels to Edges
- Directed Versus Undirected Graphs
- Reachability
- Graphs and Git
- Visualizing Your Git Repository
- References
- The Reference Reference
- Making Sense of the Display
- Garbage Collection
- Experimenting With Git
- References Make Commits Reachable
- My Humble Beginnings
- Branches as Savepoints
- Use Your Targeting Computer, Luke
- Testing Out Merges
- Rebase From the Ground Up
- Cherry-Picking Explained
- Using 'git cherry-pick' to Simulate 'git rebase'
- A Helpful Mnemonic for 'git rebase' Arguments
- The End
